Page 29 - Appplied Mathematics for the Petroleum and Other Industries, 5th Edition

188 TRIGONOMETRY

RIGHT TRIANGLE TRIGONOMETRY

Right triangle trigonometry allows you to solve problems involving triangles
that have a right angle. When you know the values of two of the sides of a right
triangle, or the value of one angle and one side of a right triangle, you can indi-
rectly calculate the values of the remaining angles and sides.
Angles are measured from an arbitrary radial line of a circle. A radial line is
a straight line that begins at the center of the circle and runs, or radiates, outward
from the center. Draw several radial lines inside a circle and the spaces between
the lines are angles. Angles are measured in degrees, minutes, and seconds. A
circle has 360 degrees (360°). Each degree is made up of 60 minutes (60') and
each minute is made up of sixty seconds (60"). Thus, a 360° circle contains
21,600' and 1,296,000".
A quadrant is one-fourth of a circle, or 90°, and a 90° angle is a right angle.
A right triangle has one right angle (a 90° angle) and two acute angles, which
are angles of less than 90°. Regardless of the size of each of the two acute angles,
their sum is 90°. Therefore, the sum of all three angles in a right triangle is 180°.
This fact can be helpful when determining the value of the acute angles in a
right triangle. For example, if you know that a right triangle has one acute angle
of 60°, you also know that the other acute angle is 30° because 180 – 90 – 60 =
30° and 90 + 60 + 30 = 180°.

B Trigonometric Functions
A trigonometric function expresses the relationship between the angles and sides
of a right triangle. Trigonometric functions, or ratios, involve two sides and an
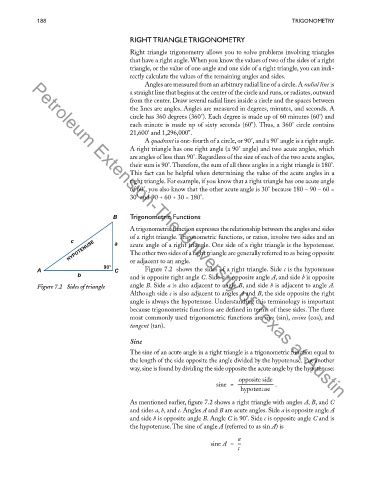
c
HYPOTENUSE a acute angle of a right triangle. One side of a right triangle is the hypotenuse.
The other two sides of a right triangle are generally referred to as being opposite

90° or adjacent to an angle.
Figure 7.2 shows the sides of a right triangle. Side c is the hypotenuse
A C
b and is opposite right angle C. Side a is opposite angle A, and side b is opposite
Figure 7.2 Sides of triangle angle B. Side a is also adjacent to angle B, and side b is adjacent to angle A.
Although side c is also adjacent to angles A and B, the side opposite the right
angle is always the hypotenuse. Understanding this terminology is important
because trigonometric functions are defined in terms of these sides. The three
most commonly used trigonometric functions are sine (sin), cosine (cos), and
tangent (tan).

Sine
The sine of an acute angle in a right triangle is a trigonometric function equal to
the length of the side opposite the angle divided by the hypotenuse. Put another
way, sine is found by dividing the side opposite the acute angle by the hypotenuse:
opposite side
sine = —————– .
Petroleum Extension-The University of Texas at Austin
hypotenuse
As mentioned earlier, figure 7.2 shows a right triangle with angles A, B, and C
and sides a, b, and c. Angles A and B are acute angles. Side a is opposite angle A
and side b is opposite angle B. Angle C is 90°. Side c is opposite angle C and is
the hypotenuse. The sine of angle A (referred to as sin A) is

a
sine A = –
c